
Luminosity is an absolute measure of radiated electromagnetic power (light), the radiant power emitted by a light-emitting object over time. In astronomy, luminosity is the total amount of electromagnetic energy emitted per unit of time by a star, galaxy, or other astronomical objects.
The Eddington luminosity, also referred to as the Eddington limit, is the maximum luminosity a body can achieve when there is balance between the force of radiation acting outward and the gravitational force acting inward. The state of balance is called hydrostatic equilibrium. When a star exceeds the Eddington luminosity, it will initiate a very intense radiation-driven stellar wind from its outer layers. Since most massive stars have luminosities far below the Eddington luminosity, their winds are mostly driven by the less intense line absorption. The Eddington limit is invoked to explain the observed luminosity of accreting black holes such as quasars.
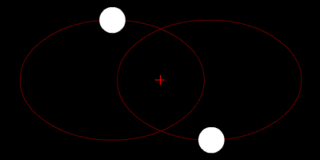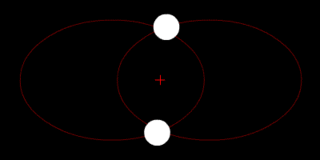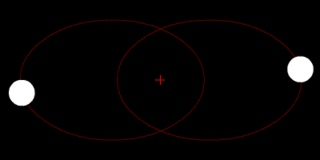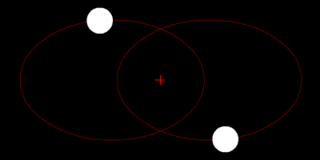
In classical mechanics, the two-body problem is to predict the motion of two massive objects which are abstractly viewed as point particles. The problem assumes that the two objects interact only with one another; the only force affecting each object arises from the other one, and all other objects are ignored.

Orbital decay is a gradual decrease of the distance between two orbiting bodies at their closest approach over many orbital periods. These orbiting bodies can be a planet and its satellite, a star and any object orbiting it, or components of any binary system. If left unchecked, the decay eventually results in termination of the orbit when the smaller object strikes the surface of the primary; or for objects where the primary has an atmosphere, the smaller object burns, explodes, or otherwise breaks up in the larger object's atmosphere; or for objects where the primary is a star, ends with incineration by the star's radiation. Collisions of stellar-mass objects are usually accompanied by effects such as gamma-ray bursts and detectable gravitational waves.

In astrodynamics or celestial mechanics, an elliptic orbit or elliptical orbit is a Kepler orbit with an eccentricity of less than 1; this includes the special case of a circular orbit, with eccentricity equal to 0. In a stricter sense, it is a Kepler orbit with the eccentricity greater than 0 and less than 1. In a wider sense, it is a Kepler orbit with negative energy. This includes the radial elliptic orbit, with eccentricity equal to 1.
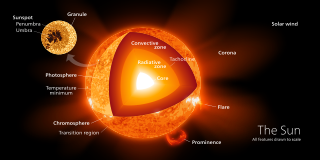
A radiation zone, or radiative region is a layer of a star's interior where energy is primarily transported toward the exterior by means of radiative diffusion and thermal conduction, rather than by convection. Energy travels through the radiation zone in the form of electromagnetic radiation as photons.
In orbital mechanics, mean motion is the angular speed required for a body to complete one orbit, assuming constant speed in a circular orbit which completes in the same time as the variable speed, elliptical orbit of the actual body. The concept applies equally well to a small body revolving about a large, massive primary body or to two relatively same-sized bodies revolving about a common center of mass. While nominally a mean, and theoretically so in the case of two-body motion, in practice the mean motion is not typically an average over time for the orbits of real bodies, which only approximate the two-body assumption. It is rather the instantaneous value which satisfies the above conditions as calculated from the current gravitational and geometric circumstances of the body's constantly-changing, perturbed orbit.
In classical mechanics, the Kepler problem is a special case of the two-body problem, in which the two bodies interact by a central force F that varies in strength as the inverse square of the distance r between them. The force may be either attractive or repulsive. The problem is to find the position or speed of the two bodies over time given their masses, positions, and velocities. Using classical mechanics, the solution can be expressed as a Kepler orbit using six orbital elements.

Gliese 667 is a triple-star system in the constellation Scorpius lying at a distance of about 7.2 parsecs from Earth. All three of the stars have masses smaller than the Sun. There is a 12th-magnitude star close to the other three, but it is not gravitationally bound to the system. To the naked eye, the system appears to be a single faint star of magnitude 5.89.

In astronomy, the initial mass function (IMF) is an empirical function that describes the initial distribution of masses for a population of stars during star formation. IMF not only describes the formation and evolution of individual stars, it also serves as an important link that describes the formation and evolution of galaxies. The IMF is often given as a probability density function (PDF) that describes the probability of a star that has a certain mass. It differs from the present-day mass function (PDMF), which describes the current distribution of masses of stars, such as red giants, white dwarfs, neutron stars, and black holes, after a period of time of evolution away from the main sequence stars. IMF is derived from the luminosity function while PDMF is derived from the present-day luminosity function. IMF and PDMF can be linked through the "stellar creation function". Stellar creation function is defined as the number of stars per unit volume of space in a mass range and a time interval. For all the main sequence stars have greater lifetimes than the galaxy, IMF and PDMF are equivalent. Similarly, IMF and PDMF are equivalent in brown dwarfs due to their unlimited lifetimes.
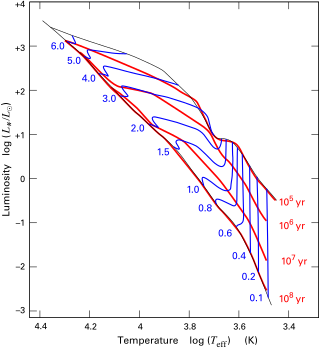
The Hayashi track is a luminosity–temperature relationship obeyed by infant stars of less than 3 M☉ in the pre-main-sequence phase of stellar evolution. It is named after Japanese astrophysicist Chushiro Hayashi. On the Hertzsprung–Russell diagram, which plots luminosity against temperature, the track is a nearly vertical curve. After a protostar ends its phase of rapid contraction and becomes a T Tauri star, it is extremely luminous. The star continues to contract, but much more slowly. While slowly contracting, the star follows the Hayashi track downwards, becoming several times less luminous but staying at roughly the same surface temperature, until either a radiative zone develops, at which point the star starts following the Henyey track, or nuclear fusion begins, marking its entry onto the main sequence.
In astronomy, the distance to a visual binary star may be estimated from the masses of its two components, the size of their orbit, and the period of their orbit about one another. A dynamical parallax is an (annual) parallax which is computed from such an estimated distance.
The two-body problem in general relativity is the determination of the motion and gravitational field of two bodies as described by the field equations of general relativity. Solving the Kepler problem is essential to calculate the bending of light by gravity and the motion of a planet orbiting its sun. Solutions are also used to describe the motion of binary stars around each other, and estimate their gradual loss of energy through gravitational radiation.
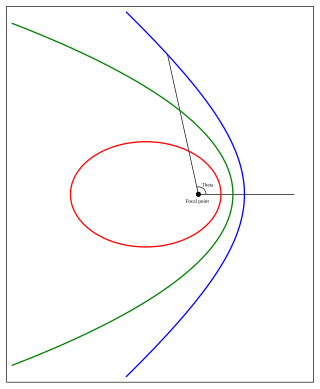
In celestial mechanics, a Kepler orbit is the motion of one body relative to another, as an ellipse, parabola, or hyperbola, which forms a two-dimensional orbital plane in three-dimensional space. A Kepler orbit can also form a straight line. It considers only the point-like gravitational attraction of two bodies, neglecting perturbations due to gravitational interactions with other objects, atmospheric drag, solar radiation pressure, a non-spherical central body, and so on. It is thus said to be a solution of a special case of the two-body problem, known as the Kepler problem. As a theory in classical mechanics, it also does not take into account the effects of general relativity. Keplerian orbits can be parametrized into six orbital elements in various ways.

In geometry, the major axis of an ellipse is its longest diameter: a line segment that runs through the center and both foci, with ends at the two most widely separated points of the perimeter. The semi-major axis is the longest semidiameter or one half of the major axis, and thus runs from the centre, through a focus, and to the perimeter. The semi-minor axis of an ellipse or hyperbola is a line segment that is at right angles with the semi-major axis and has one end at the center of the conic section. For the special case of a circle, the lengths of the semi-axes are both equal to the radius of the circle.
In astrophysics, the mass–luminosity relation is an equation giving the relationship between a star's mass and its luminosity, first noted by Jakob Karl Ernst Halm. The relationship is represented by the equation:
In astrophysics the chirp mass of a compact binary system determines the leading-order orbital evolution of the system as a result of energy loss from emitting gravitational waves. Because the gravitational wave frequency is determined by orbital frequency, the chirp mass also determines the frequency evolution of the gravitational wave signal emitted during a binary's inspiral phase. In gravitational wave data analysis it is easier to measure the chirp mass than the two component masses alone.
HD 98649 is a G-type yellow dwarf star, classified as a G4V, that has approximately the same mass and diameter as the Sun, but has only 86% of its luminosity. It is considered a solar analog. HD 98649 is about 138 light-years from earth. HD 98649 is found in the Crater constellation.
In astronomy, the binary mass function or simply mass function is a function that constrains the mass of the unseen component in a single-lined spectroscopic binary star or in a planetary system. It can be calculated from observable quantities only, namely the orbital period of the binary system, and the peak radial velocity of the observed star. The velocity of one binary component and the orbital period provide information on the separation and gravitational force between the two components, and hence on the masses of the components.

Kepler-1229b is a confirmed super-Earth exoplanet, likely rocky, orbiting within the habitable zone of the red dwarf Kepler-1229, located about 870 light years from Earth in the constellation of Cygnus. It was discovered in 2016 by the Kepler space telescope. The exoplanet was found by using the transit method, in which the dimming effect that a planet causes as it crosses in front of its star is measured.


























































