
In calculus, an antiderivative, inverse derivative, primitive function, primitive integral or indefinite integral of a function f is a differentiable function F whose derivative is equal to the original function f. This can be stated symbolically as F' = f. The process of solving for antiderivatives is called antidifferentiation, and its opposite operation is called differentiation, which is the process of finding a derivative. Antiderivatives are often denoted by capital Roman letters such as F and G.
In complex analysis, an entire function, also called an integral function, is a complex-valued function that is holomorphic on the whole complex plane. Typical examples of entire functions are polynomials and the exponential function, and any finite sums, products and compositions of these, such as the trigonometric functions sine and cosine and their hyperbolic counterparts sinh and cosh, as well as derivatives and integrals of entire functions such as the error function. If an entire function has a root at , then , taking the limit value at , is an entire function. On the other hand, the natural logarithm, the reciprocal function, and the square root are all not entire functions, nor can they be continued analytically to an entire function.
In mathematics, an elementary function is a function of a single variable that is defined as taking sums, products, roots and compositions of finitely many polynomial, rational, trigonometric, hyperbolic, and exponential functions, including possibly their inverse functions.

In mathematics, an integral is the continuous analog of a sum, which is used to calculate areas, volumes, and their generalizations. Integration, the process of computing an integral, is one of the two fundamental operations of calculus, the other being differentiation. Integration was initially used to solve problems in mathematics and physics, such as finding the area under a curve, or determining displacement from velocity. Usage of integration expanded to a wide variety of scientific fields thereafter.

The natural logarithm of a number is its logarithm to the base of the mathematical constant e, which is an irrational and transcendental number approximately equal to 2.718281828459. The natural logarithm of x is generally written as ln x, logex, or sometimes, if the base e is implicit, simply log x. Parentheses are sometimes added for clarity, giving ln(x), loge(x), or log(x). This is done particularly when the argument to the logarithm is not a single symbol, so as to prevent ambiguity.
In mathematics, a transcendental number is a real or complex number that is not algebraic – that is, not the root of a non-zero polynomial of finite degree with rational coefficients. The best-known transcendental numbers are π and e.

In analysis, numerical integration comprises a broad family of algorithms for calculating the numerical value of a definite integral. The term numerical quadrature is more or less a synonym for "numerical integration", especially as applied to one-dimensional integrals. Some authors refer to numerical integration over more than one dimension as cubature; others take "quadrature" to include higher-dimensional integration.
In mathematics, an irreducible polynomial is, roughly speaking, a polynomial that cannot be factored into the product of two non-constant polynomials. The property of irreducibility depends on the nature of the coefficients that are accepted for the possible factors, that is, the field to which the coefficients of the polynomial and its possible factors are supposed to belong. For example, the polynomial x2 − 2 is a polynomial with integer coefficients, but, as every integer is also a real number, it is also a polynomial with real coefficients. It is irreducible if it is considered as a polynomial with integer coefficients, but it factors as if it is considered as a polynomial with real coefficients. One says that the polynomial x2 − 2 is irreducible over the integers but not over the reals.
Integration is the basic operation in integral calculus. While differentiation has straightforward rules by which the derivative of a complicated function can be found by differentiating its simpler component functions, integration does not, so tables of known integrals are often useful. This page lists some of the most common antiderivatives.
Axiom is a free, general-purpose computer algebra system. It consists of an interpreter environment, a compiler and a library, which defines a strongly typed hierarchy.
In mathematics, an expression is in closed form if it is formed with constants, variables and a finite set of basic functions connected by arithmetic operations and function composition. Commonly, the allowed functions are nth root, exponential function, logarithm, and trigonometric functions. However, the set of basic functions depends on the context.
In mathematics, a nonelementary antiderivative of a given elementary function is an antiderivative that is, itself, not an elementary function. A theorem by Liouville in 1835 provided the first proof that nonelementary antiderivatives exist. This theorem also provides a basis for the Risch algorithm for determining which elementary functions have elementary antiderivatives.
In mathematics, the Bernstein–Sato polynomial is a polynomial related to differential operators, introduced independently by Joseph Bernstein and Mikio Sato and Takuro Shintani, Sato (1990). It is also known as the b-function, the b-polynomial, and the Bernstein polynomial, though it is not related to the Bernstein polynomials used in approximation theory. It has applications to singularity theory, monodromy theory, and quantum field theory.
In calculus, symbolic integration is the problem of finding a formula for the antiderivative, or indefinite integral, of a given function f(x), i.e. to find a formula for a differentiable function F(x) such that

In applied mathematics, a transcendental equation is an equation over the real numbers that is not algebraic, that is, if at least one of its sides describes a transcendental function. Examples include:
In mathematics, Liouville's theorem, originally formulated by Joseph Liouville in 1833 to 1841, places an important restriction on antiderivatives that can be expressed as elementary functions.

SymPy is an open-source Python library for symbolic computation. It provides computer algebra capabilities either as a standalone application, as a library to other applications, or live on the web as SymPy Live or SymPy Gamma. SymPy is simple to install and to inspect because it is written entirely in Python with few dependencies. This ease of access combined with a simple and extensible code base in a well known language make SymPy a computer algebra system with a relatively low barrier to entry.
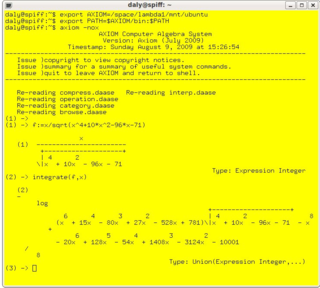
In mathematics and computer science, computer algebra, also called symbolic computation or algebraic computation, is a scientific area that refers to the study and development of algorithms and software for manipulating mathematical expressions and other mathematical objects. Although computer algebra could be considered a subfield of scientific computing, they are generally considered as distinct fields because scientific computing is usually based on numerical computation with approximate floating point numbers, while symbolic computation emphasizes exact computation with expressions containing variables that have no given value and are manipulated as symbols.
In mathematics, the Liouvillian functions comprise a set of functions including the elementary functions and their repeated integrals. Liouvillian functions can be recursively defined as integrals of other Liouvillian functions.
Most of the terms listed in Wikipedia glossaries are already defined and explained within Wikipedia itself. However, glossaries like this one are useful for looking up, comparing and reviewing large numbers of terms together. You can help enhance this page by adding new terms or writing definitions for existing ones.















